Learning Objectives
- Explain how living organisms maintain order without violating the second law of thermodynamics
- Predict the direction of reactions from Gibbs free energy changes, and vice versa
- Distinguish between steady-state (homeostasis) and chemical equilibrium with respect to bioenergetics
- Use energy diagrams to explain how catalysts increase rates of reaction
- Interpret enzyme kinetics graphs using Km and Vmax
- Define allosteric regulation, and distinguish between competitive and noncompetitive inhibition
How do the laws of thermodynamics apply to living organisms?
- The First Law says that energy cannot be created or destroyed.
- The Second Law says that in any energy conversion, some energy is wasted as heat; moreover, the entropy of any closed system always increases.
One way we can see the Second Law at work is in our daily diet. We eat food each day, without gaining that same amount of body weight! The food we eat is largely expended as carbon dioxide and heat energy, plus some work done in repairing and rebuilding bodily cells and tissues, physical movement, and neuronal activity.
Although living organisms appear to reduce entropy, by assembling small molecules into polymers and higher order structures, this work releases waste heat that increases the entropy of the environment.
Gibbs Free Energy
Gibbs free energy is a measure of the amount of work that is potentially obtainable. Instead of absolute quantities, what is usually measured is the change in free energy in the course of a reaction:
ΔG = ΔH – TΔS
where H = enthalpy (a measure of energy content that is roughly equivalent to heat content), T = absolute temperature (Kelvin), and S = entropy. Entropy, while sometimes called ‘disorder,’ is a complicated and subtle concept that has more to do with degrees of freedom for how molecules are organized: the same 6 molecules of carbon in CO2 have greater entropy than they do in a molecule of glucose, where the carbon atoms are linked together by covalent bonds.
If ΔG < 0, a chemical reaction is exergonic, releases free energy, and can progress spontaneously, with no input of additional energy. This negative ΔG does not mean that the reaction will occur quickly, as we’ll see the discussion about reaction rates below.
If ΔG > 0, a chemical reaction is endergonic, requires or absorbs an input of free energy, and will progress only if free energy is put into the system. Without that infusion of free energy, the reaction will go backwards.
Free energy and chemical equilibrium
If ΔG = 0, a chemical reaction is in equilibrium, meaning that the rates of forward and reverse reactions are equal, so there is no net change, or no potential for doing work. In biological systems, more often systems are not at equilibrium. Instead, reactions proceed spontaneously towards equilibrium, depending on whether the products or reactants are more available and whether the reaction is chemically favorable. The equilibrium point (labled eq in the figure below) is the minimum free energy state of the reaction mixture.

Cells couple exergonic reactions to endergonic reactions so that the net free energy change is negative
ATP is the primary energy currency of the cell. Cells use ATP to accomplish endergonic reactions such as active transport, cell movement, or protein synthesis by tapping the energy of ATP hydrolysis:
ATP -> ADP + Pi (Pi = PO4, inorganic phosphate); ΔG = -7.3 kcal/mol
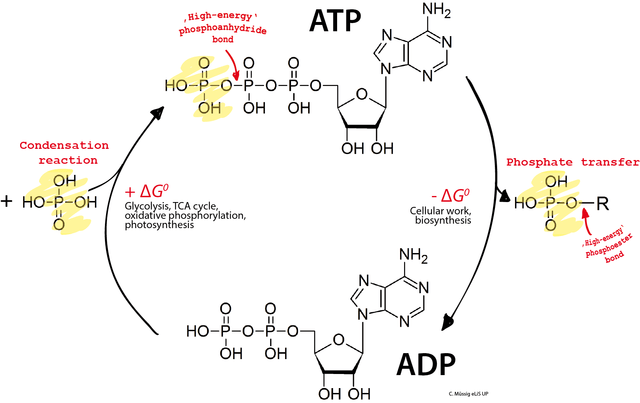
Over the next pages we’ll be looking at the cellular metabolic pathways that phosphorylate ADP to make ATP.
Reaction rates
Although the sign of ΔG (negative or positive) determines the direction that the reaction will go spontaneously, the magnitude of ΔG does not predict how fast the reaction will go.
The rate of the reaction is determined by the activation energy (the energy required to attain the transition state) barrier Ea:
The peak of this energy diagram represents the transition state: an intermediate stage in the reaction from which the reaction can go in either direction. Reactions with a high activation energy will proceed very slowly because only a few molecules will obtain enough energy to reach the transition state—even if they are highly exergonic. In the figure above, the reaction from X->Y has a much greater activation energy than the reverse reaction Y->X. Starting with equal amounts of X and Y, the reaction will go in reverse.
The addition of a catalyst, an agent that speeds up the rate of a reaction but is not consumed or altered by the reaction, provides an alternative transition state with lower activation energy. What this means is that the catalyst holds the substrates in a physical conformation that makes the reaction more likely to proceed. As a result, in the presence of the catalyst, a much higher percentage of molecules (X or Y) can acquire enough energy to attain the transition state, so the reaction can go faster in either direction. Note that the catalyst only affects the energy of the transition state and does not affect the overall free energy change of the reaction. This means that reactions with a catalyst go faster but do not have different free energy change.
Enzymes speed up reactions by lowering the activation energy barrier
Enzymes are biological catalysts, and therefore not consumed or altered by the reactions they catalyze. They repeatedly bind substrate to convert and release product. They do this for as long as substrate molecules are available and thermodynamic conditions are favorable, when ΔG is negative and the product/substrate ratio is lower than the equilibrium ratio. Most enzymes are proteins, but several key enzymes are RNA molecules (ribozymes). Enzymes are highly specific for their substrates. Only molecules with a particular shape and chemical groups in the right positions can interact with amino acid side chains at the active site, which is the substrate-binding site of the enzyme.
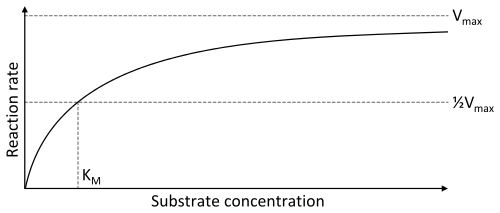
Enzyme-catalyzed reactions have saturation kinetics
The velocity of enzyme-catalyzed reactions increases with the concentration of substrate. However, at high substrate concentrations, the quantity of enzyme molecules becomes limiting as every enzyme molecule is working as fast as it can. At saturation, further increases in substrate concentrations have no effect; the only way to increase reaction rates is to increase the amount of enzyme.
The kinetic properties of enzymes are defined by their Vmax and Km
- The Vmax is the maximum rate at which enzymes can work, and represents work at saturating concentrations of substrate.
- The Km (Michaelis constant) is defined as the substrate concentration that produces a rate equal to 1/2 Vmax and is a measure of the affinity of the enzyme for its substrate.
Enzyme inhibitors
Enzymes are subject to regulation, and are the targets of many pharmaceutical drugs, such as non-steroidal pain relievers. Many enzymes are regulated by allosteric regulators which bind at a site distinct from the active site.
Noncompetitive inhibitors act allosterically (bind at a site different from the active site). When the noncompetitive inhibitor binds allosterically, it often changes the overall shape of the enzyme, including the active site, so that substrates can no longer bind to the active site.
Competitive inhibitors compete with the substrate for binding to the active site; the enzyme cannot carry out its normal reaction with the inhibitor, because the inhibitor physically blocks the substrate from binding the active site.
Below are lecture videos on thermodynamics and enzymes (with apologies for the audio lag).
Powerpoint file used in the videos: free_energy
Sustainable Development Goals

UN Sustainable Development Goal (SDG) 11: Sustainable Cities and Communities – The ability to catalyze and predict the direction of spontaneous reactions is useful in understanding the thermodynamics of energy conversion processes. Such processes can be optimized for efficiency and reduced waste/pollution, and can be engineered for resiliency in the face of natural disasters, rendering such technologies more sustainable and scalable in the long-term, particularly as cities expand. Furthermore, more nuanced understandings of activation sites and inhibition can be used to more effectively control disease-carrying pest populations in urban areas, making for more sustainable solutions to human-wildlife interactions as areas grow more populated.